Multiple Root
Pronunciation: /ˈmʌl.tə.pəl rut/ Explain
|
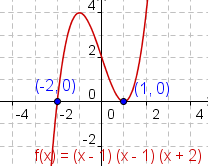
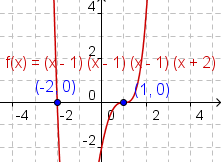
A multiple root of a polynomial is a root that occurs more than once.[2] Take the polynomial in figure 1, (x - 1)(x - 1)(x + 2) The factor (x - 1) occurs twice, so it is a multiple root. Since it occurs only twice, it is also called a double root. The graph of polynomials with a double root just touches the x-axis at the root then changes direction. Figure 2 shows the graph of the polynomial (x - 1)(x - 1)(x - 1)(x + 2). In this polynomial, the term (x - 1) occurs 3 times. It has a multiplicity of 3. It is also called a triple root. Note that the graph of the polynomial crosses the x-axis at the triple root. |
|
Discovery
|
|
References
- McAdams, David E.. All Math Words Dictionary, multiple root. 2nd Classroom edition 20150108-4799968. pg 120. Life is a Story Problem LLC. January 8, 2015. Buy the book
- Dickson, Leonard Eugene, Ph.D.. First Course in the Theory of Equations. ch II pg 18. New York, John Wiley and Sons Inc.. 1922. Last Accessed 9/2/2018. http://www.gutenberg.org/files/29785/29785-pdf.pdf. Buy the book
Cite this article as:
McAdams, David E. Multiple Root. 4/25/2019. All Math Words Encyclopedia. Life is a Story Problem LLC. https://www.allmathwords.org/en/m/multipleroot.html.Image Credits
- All images and manipulatives are by David McAdams unless otherwise stated. All images by David McAdams are Copyright © Life is a Story Problem LLC and are licensed under a Creative Commons Attribution-ShareAlike 4.0 International License.
Revision History
4/25/2019: Changed equations and expressions to new format. (McAdams, David E.)12/21/2018: Reviewed and corrected IPA pronunication. (McAdams, David E.)
9/4/2018: Removed broken links, updated license, implemented new markup. (McAdams, David E.)
12/18/2009: Added revision. (McAdams, David E.)
12/16/2008: Initial version. (McAdams, David E.)
- Navigation
- Home
- Contents
-
# A B C D E F G H I J K L M N O P Q R S T U V W X Y Z - Teacher Aids
- Classroom Demos
- How To
- LIASP
- LIASP Home
- Conditions of Use
- Privacy Policy
- Donate to LIASP
- Help build this site
- About LIASP
- Contact LIASP
All Math Words Encyclopedia is a service of
Life is a Story Problem LLC.
Copyright © 2018 Life is a Story Problem LLC. All rights reserved.
This work is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License